The Yeoh hyperelastic material model[1] is a phenomenological model for the deformation of nearly incompressible, nonlinear elastic materials such as rubber. The model is based on Ronald Rivlin's observation that the elastic properties of rubber may be described using a strain energy density function which is a power series in the strain invariants of the Cauchy-Green deformation tensors.[2] The Yeoh model for incompressible rubber is a function only of . For compressible rubbers, a dependence on is added on. Since a polynomial form of the strain energy density function is used but all the three invariants of the left Cauchy-Green deformation tensor are not, the Yeoh model is also called the reduced polynomial model.
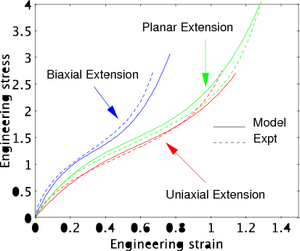
Yeoh model for incompressible rubbers
editStrain energy density function
editThe original model proposed by Yeoh had a cubic form with only dependence and is applicable to purely incompressible materials. The strain energy density for this model is written as
where are material constants. The quantity can be interpreted as the initial shear modulus.
Today a slightly more generalized version of the Yeoh model is used.[3] This model includes terms and is written as
When the Yeoh model reduces to the neo-Hookean model for incompressible materials.
For consistency with linear elasticity the Yeoh model has to satisfy the condition
where is the shear modulus of the material. Now, at ,
Therefore, the consistency condition for the Yeoh model is
Stress-deformation relations
editThe Cauchy stress for the incompressible Yeoh model is given by
Uniaxial extension
editFor uniaxial extension in the -direction, the principal stretches are . From incompressibility . Hence . Therefore,
The left Cauchy-Green deformation tensor can then be expressed as
If the directions of the principal stretches are oriented with the coordinate basis vectors, we have
Since , we have
Therefore,
The engineering strain is . The engineering stress is
Equibiaxial extension
editFor equibiaxial extension in the and directions, the principal stretches are . From incompressibility . Hence . Therefore,
The left Cauchy-Green deformation tensor can then be expressed as
If the directions of the principal stretches are oriented with the coordinate basis vectors, we have
Since , we have
Therefore,
The engineering strain is . The engineering stress is
Planar extension
editPlanar extension tests are carried out on thin specimens which are constrained from deforming in one direction. For planar extension in the directions with the direction constrained, the principal stretches are . From incompressibility . Hence . Therefore,
The left Cauchy-Green deformation tensor can then be expressed as
If the directions of the principal stretches are oriented with the coordinate basis vectors, we have
Since , we have
Therefore,
The engineering strain is . The engineering stress is
Yeoh model for compressible rubbers
editA version of the Yeoh model that includes dependence is used for compressible rubbers. The strain energy density function for this model is written as
where , and are material constants. The quantity is interpreted as half the initial shear modulus, while is interpreted as half the initial bulk modulus.
When the compressible Yeoh model reduces to the neo-Hookean model for incompressible materials.
History
editThe model is named after Oon Hock Yeoh. Yeoh completed his doctoral studies under Graham Lake at the University of London.[4] Yeoh held research positions at Freudenberg-NOK, MRPRA (England), Rubber Research Institute of Malaysia (Malaysia), University of Akron, GenCorp Research, and Lord Corporation.[5] Yeoh won the 2004 Melvin Mooney Distinguished Technology Award from the ACS Rubber Division.[6]
References
edit- ^ Yeoh, O. H. (November 1993). "Some forms of the strain energy function for rubber". Rubber Chemistry and Technology. 66 (5): 754–771. doi:10.5254/1.3538343.
- ^ Rivlin, R. S., 1948, "Some applications of elasticity theory to rubber engineering", in Collected Papers of R. S. Rivlin vol. 1 and 2, Springer, 1997.
- ^ Selvadurai, A. P. S., 2006, "Deflections of a rubber membrane", Journal of the Mechanics and Physics of Solids, vol. 54, no. 6, pp. 1093-1119.
- ^ "Remembering Dr. Graham Johnson Lake (1935–2023)". Rubber Chemistry and Technology. 96 (4): G2 – G3. 2023. doi:10.5254/rct-23.498080.
- ^ "Biographical Sketch". ACS Rubber Division. Retrieved 20 February 2024.
- ^ "Rubber Division names 3 for awards". Rubber and Plastics News. Crain. 27 October 2003. Retrieved 16 August 2022.


